Welcome to the Half Life And Rate Of Decay MDCAT MCQs with Answers. In this post, we have shared Half Life And Rate Of Decay Multiple Choice Questions and Answers for PMC MDCAT 2024. Each question in MDCAT Physics offers a chance to enhance your knowledge regarding Half Life And Rate Of Decay MCQs in this MDCAT Online Test.
Half Life And Rate Of Decay MDCAT MCQs Test Preparations
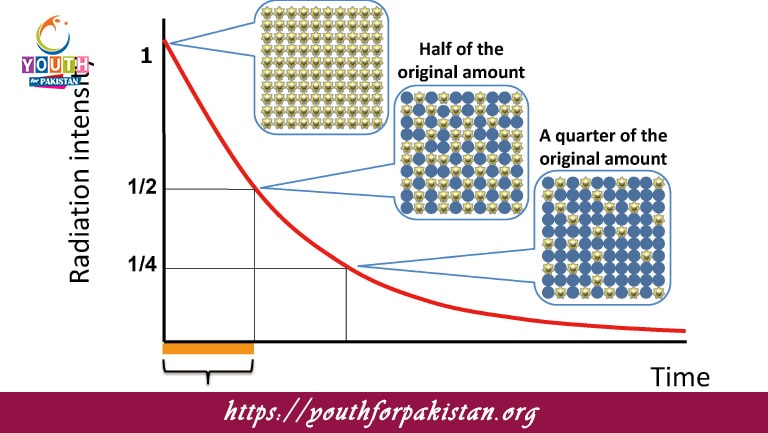
What is the half-life of a radioactive substance?
A) The time taken for the substance to completely decay
B) The time taken for half the nuclei to decay
C) The time taken for a quarter of the substance to decay
D) The time taken for all radiation to be emitted
If the half-life of a radioactive isotope is 5 years, what fraction of the original sample remains after 15 years?
A) 1/4
B) 1/8
C) 1/16
D) 1/32
A sample initially has 160 grams of a radioactive isotope. After 3 half-lives, how much of the sample remains?
A) 20 grams
B) 40 grams
C) 10 grams
D) 5 grams
The decay constant (λ) of a radioactive isotope is related to its half-life (T½) by the formula:
A) λ = ln(2) / T½
B) λ = T½ / ln(2)
C) λ = 2 ln(T½)
D) λ = T½ / 2
A radioactive isotope decays with a half-life of 8 days. What is the fraction of the original activity remaining after 16 days?
A) 1/2
B) 1/3
C) 1/4
D) 1/8
The half-life of Carbon-14 is approximately 5730 years. How much Carbon-14 remains after 11,460 years?
A) 1/2
B) 1/4
C) 1/8
D) 1/16
A sample of a radioactive substance has an initial activity of 200 Bq. After 3 half-lives, what will the activity be?
A) 25 Bq
B) 50 Bq
C) 100 Bq
D) 12.5 Bq
The rate of decay of a radioactive isotope is:
A) Proportional to the mass of the isotope
B) Proportional to the number of undecayed nuclei
C) Independent of the amount of substance
D) Proportional to the energy emitted
If a radioactive element has a half-life of 2 years, how long will it take for 75% of the sample to decay?
A) 1 year
B) 2 years
C) 3 years
D) 4 years
A radioactive sample decays from 80 grams to 10 grams in 12 hours. What is the half-life of the substance?
A) 2 hours
B) 3 hours
C) 4 hours
D) 6 hours
The concept of half-life can be applied to:
A) Only radioactive decay
B) Both radioactive decay and chemical reactions
C) Only chemical reactions
D) Only biological processes
If a sample’s activity drops to one-sixteenth of its original value, how many half-lives have passed?
A) 2
B) 3
C) 4
D) 5
The half-life of a radioactive substance is 10 hours. If you start with 1000 atoms, how many atoms will remain undecayed after 20 hours?
A) 250
B) 500
C) 125
D) 100
Which of the following does NOT affect the half-life of a radioactive isotope?
A) Temperature
B) Pressure
C) Chemical form
D) All of the above
The activity of a radioactive sample decreases over time due to:
A) Decrease in the number of radioactive nuclei
B) Increase in the energy of emitted particles
C) Absorption of radiation by the sample
D) Emission of gamma rays
If the decay constant of a radioactive substance is 0.693 per day, what is its half-life?
A) 1 day
B) 0.5 day
C) 0.693 day
D) 2 days
The half-life of a substance is inversely proportional to:
A) The decay constant
B) The atomic number
C) The activity
D) The number of nuclei
What percentage of a radioactive sample decays after 3 half-lives?
A) 12.5%
B) 25%
C) 75%
D) 87.5%
A sample of a radioactive isotope decays to 1/8th of its original amount in 18 hours. What is the half-life of the isotope?
A) 3 hours
B) 6 hours
C) 9 hours
D) 12 hours
The term “mean life” of a radioactive isotope refers to:
A) The average time taken for all nuclei to decay
B) The time at which the activity becomes zero
C) The average time taken for a single nucleus to decay
D) The time taken for half of the sample to decay
After 5 half-lives, what fraction of the original radioactive substance remains?
A) 1/2
B) 1/5
C) 1/16
D) 1/32
What is the relationship between the activity (A) of a sample and the number of undecayed nuclei (N)?
A) A = λN
B) A = λ/N
C) A = N/λ
D) A = N/2
If a radioactive sample has a half-life of 24 hours, what fraction of the sample remains after 72 hours?
A) 1/8
B) 1/4
C) 1/3
D) 1/16
The decay of a radioactive substance is described by the equation N = N₀e^(-λt). What does N represent?
A) The initial number of nuclei
B) The number of undecayed nuclei at time t
C) The decay constant
D) The half-life
If a sample’s activity decreases from 800 Bq to 100 Bq in 12 hours, what is the decay constant (λ)?
A) 0.0578 per hour
B) 0.1156 per hour
C) 0.2312 per hour
D) 0.4624 per hour
The number of remaining nuclei of a radioactive sample can be calculated using which formula?
A) N = N₀e^(λt)
B) N = N₀e^(-λt)
C) N = N₀ / e^(λt)
D) N = N₀ / e^(-λt)
A radioactive substance has a half-life of 15 years. What percentage of the substance will remain after 45 years?
A) 12.5%
B) 25%
C) 6.25%
D) 3.125%
If the initial number of nuclei in a radioactive sample is 5000 and its half-life is 4 hours, how many nuclei will remain after 12 hours?
A) 625
B) 250
C) 125
D) 50
The decay rate of a radioactive sample is defined as:
A) The number of decays per unit time
B) The time taken for half of the nuclei to decay
C) The total number of remaining nuclei
D) The initial activity of the sample
What is the mean life (τ) of a radioactive substance if its half-life is 8 hours?
A) 4 hours
B) 8 hours
C) 16 hours
D) 11.2 hours
If the decay constant (λ) of a substance is 0.1 per year, what is its half-life?
A) 6.93 years
B) 10 years
C) 7.77 years
D) 5 years
How many grams of a radioactive substance will remain after 2 half-lives if you start with 64 grams?
A) 16 grams
B) 32 grams
C) 8 grams
D) 4 grams
Which of the following correctly represents the relationship between the decay constant (λ) and the half-life (T½)?
A) λ = T½ / ln(2)
B) λ = ln(2) / T½
C) λ = T½ / 2
D) λ = ln(2) × T½
After how many half-lives will only 1/16 of the original amount of a radioactive isotope remain?
A) 3 half-lives
B) 4 half-lives
C) 5 half-lives
D) 6 half-lives
The fraction of a radioactive sample that decays in a time period equal to its half-life is:
A) 1/4
B) 1/2
C) 1/8
D) 3/4
What is the formula for calculating the remaining quantity of a substance after a given time?
A) Q = Q₀(1/2)^(t/T½)
B) Q = Q₀e^(λt)
C) Q = Q₀e^(-λt)
D) Q = Q₀ / (1/2)^(t/T½)
How is the activity (A) of a radioactive sample related to the decay constant (λ) and the number of nuclei (N)?
A) A = λN
B) A = N / λ
C) A = λ / N
D) A = N * λ^2
A radioactive isotope has a half-life of 6 hours. What is the remaining activity if it started with 1000 Bq after 18 hours?
A) 125 Bq
B) 250 Bq
C) 500 Bq
D) 62.5 Bq
The mean life (τ) of a radioactive isotope is 15 hours. What is its half-life?
A) 7.5 hours
B) 10 hours
C) 20 hours
D) 30 hours
If the initial number of radioactive nuclei is 2000 and its half-life is 10 minutes, how many nuclei remain after 30 minutes?
A) 250
B) 500
C) 1000
D) 750
The half-life of a radioactive element is:
A) The time required for the sample to reach zero activity
B) The time required for the sample to decay to one-fourth of its original amount
C) The time required for the sample’s activity to become half
D) The time required for the sample to decay to one-half of its original quantity
The decay constant (λ) and mean life (τ) are related by the equation:
A) τ = 1 / λ
B) τ = λ / 2
C) τ = λ / ln(2)
D) τ = 2λ
If a radioactive substance has a half-life of 3 days, what fraction of the substance will remain after 9 days?
A) 1/3
B) 1/8
C) 1/9
D) 1/27
The rate of decay of a radioactive sample is proportional to:
A) The square of the number of undecayed nuclei
B) The square root of the number of undecayed nuclei
C) The number of undecayed nuclei
D) The total mass of the sample
If you are interested to enhance your knowledge regarding Physics, Chemistry, Computer, and Biology please click on the link of each category, you will be redirected to dedicated website for each category.